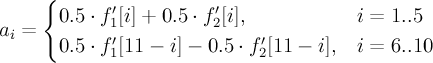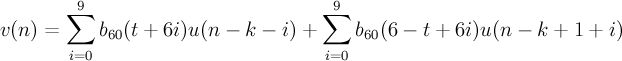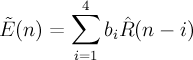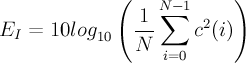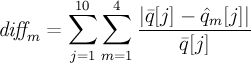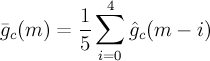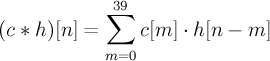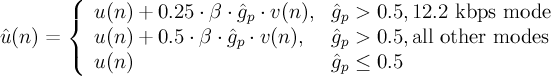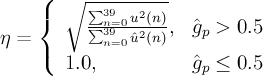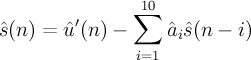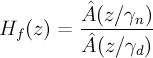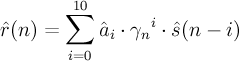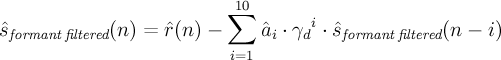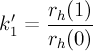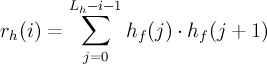AMR-NB

samples: http://samples.mplayerhq.hu/A-codecs/amr/
specification: http://www.3gpp.org/ftp/Specs/html-info/26-series.htm
Wikipedia article: http://en.wikipedia.org/wiki/Adaptive_Multi-Rate

AMR-NB (Adaptive Multi-Rate Narrowband) is a vocoder employed in low-bitrate applications like mobile phones. It is a form of ACELP where A stands for algebraic.

The following text aims to be a simpler and more explicit document of the AMR narrow band decoding processes to aid in development of a decoder. Reference to sections of the specification will be made in the following format: (c.f. §5.2.5). Happy reading.

Nomenclature weirdness

Throughout the specification, a number of references are made to the same (or very similar) items with fairly confusing variation. They are listed below to aid understanding of the following text but efforts will be made to consistently use one item name throughout or to use both with the lesser used name in parenthesis.

Pitch / Adaptive codebook
Fixed / Innovative (also algebraic when referring to the codebook)
Quantified means quantized

Summary

Mode dependent bitstream parsing
Indices parsed from bitstream
Indices decoded to give LSF vectors, fractional pitch lags, innovative code vectors and the pitch and innovative gains
LSF vectors converted to LP filter coefficients at each subframe
Subframe decoding
- Excitation vector = adaptive code vector * adaptive (pitch) gain + innovative code vector * innovative gain
- Excitation vector filtered through an LP synthesis filter to reconstruct speech
- Speech signal filtered with adaptive postfilter

Bit stream frame format

Specification (26.101) describes two possible frame types - interface formats 1 and 2 (often abbreviated IF1 and IF2). IF2 is byte-aligned. The following tables of data are taken from this specification unless otherwise stated. AMR-NB over GSM uses a third slightly different format whose bit-packing is tightly defined by the GSM specs.

IF1 format

bits	low level meaning	high level meaning
4	Frame type	AMR header
1	Frame quality indicator (0 bad/1 good)	AMR header
3	Mode indication	AMR auxiliary information
3	Mode request
8	CRC
	Class A bits	AMR core frame
	Class B bits
	Class C bits

IF2 format

bits	low level meaning	high level meaning
4	Frame type	AMR header
	Class A bits	AMR core frame
	Class B bits
	Class C bits
	Padding (called "Bit stuffing" in the specification)

GSM OTA format

bits	low level meaning	high level meaning
4	Mode request	AMR auxiliary information
1	F bit (0 good/1 unusual)	AMR header
4	Frame Type
1	Frame quality indicator (0 bad/1 good)
	Class A bits	AMR core frame
6	CRC
	Class B bits

Field meaning

Frame type	Frame content
0	AMR 4.75kbps
1	AMR 5.15kbps
2	AMR 5.90kbps
3	AMR 6.70kbps (PDC-EFR)
4	AMR 7.40kbps (TDMA-EFR)
5	AMR 7.95kbps
6	AMR 10.2kbps
7	AMR 12.2kbps (GSM-EFR)
8	AMR SID
9	GSM-EFR SID
10	TDMA-EFR SID
11	PDC-EFR SID
12-14	Reserved for future use
15	No data (no transmission/no reception)

Classes

Class	Importance explanation
A	Data that is most sensitive to error. Any error in these bits leads to a corrupted speech frame that should not be decoded without appropriate error concealment. This class of bits is protected by an 8-bit CRC.
B	Less sensitive data that are present in all speech frames.
C	Least sensitive data present only in higher bit rate frames.

Class A is protected by an 8-bit CRC with polynomial x^8+x^6+x^5+x^4+1 computed over the Class A bits.
However for AMR-NB over GSM the CRC is a 6-bit with polynomial x^6+x^5+x^3+x^2+x+1 computed over the Class A bits, and it immediately follows the Class A bits, rather than preceding them. That is, it's between the Class A and Class B bits. [see 3GPP TS 05.03 sec 3.9.4.3]
There is no significant step-wise change in subjective importance at class boundaries.
The distribution of bits is ordered from most to least subjective importance at both the class level and within the classes.

Frame type	Total bits	Class A bits	Class B bits	Class C bits
0	95	42	53	0
1	103	49	54	0
2	118	55	63	0
3	134	58	76	0
4	148	61	87	0
5	159	75	84	0
6	204	65	99	40
7	244	81	103	60
8	39	39	0	0
9	43	43	0	0
10	38	38	0	0
11	37	37	0	0

For the specifics of the bit stream layout (i.e. for the bits to parameter mappings) see 26.101 AMR speech codec frame structure and 26.090 AMR speech codec transcoding functions.

Decoding of LP filter parameters

The received indices of LSP quantization are used to reconstruct the quantified LSP vectors. (c.f. §5.2.5)

12.2kbps mode summary

indices into code books are parsed from the bit stream
indices give elements of split matrix quantised (SMQ) residual LSF vectors from the relevant code books
prediction from the previous frame is added to obtain the mean-removed LSF vectors
the mean is added
the LSF vectors are converted to cosine domain LSP vectors

Decoding SMQ residual LSF vectors

The elements of the SMQ vectors are stored at an index into a code book that varies according to the mode. There are 5 code books for the 12.2kbps mode corresponding to the 5 indices. These tables will be referred to as:

lsf_m_n

m: the number of indices parsed according to the mode
n: the index 'position' i.e. 1 for the first index, etc

The 5 indices are stored using 7, 8, 8 + sign bit, 8, 6 bits respectively. The four elements of a 'split quantized sub-matrix' are stored at the index position in the appropriate code book are reassigned as follows:

lsf_5_1[index1]: r1_1, r1_2, r2_1, r2_2
lsf_5_2[index2]: r1_3, r1_4, r2_3, r2_4
lsf_5_3[index3]: r1_5, r1_6, r2_5, r2_6
lsf_5_4[index4]: r1_7, r1_8, r2_7, r2_8
lsf_5_5[index5]: r1_9, r1_10, r2_9, r2_10

With rj_i :

j: the first or second residual lsf vector
i: the coefficient of a residual lsf vector ( i = 1, ..., 10 )

rj_i: residual line spectral frequencies (LSFs) in Hz

Mean-removed LSF vector prediction

z_j(n): the mean-removed LSF vector at the jth subframe
r_j(n): prediction residual vector of frame n at the jth subframe
^r_2(n-1): the quantified residual vector from the previous frame at the 2nd subframe

The mean is added

lsf_mean_m: a table of the means of the LSF coefficients
m: the number of indices parsed according to the mode
fj: the LSF vectors

LSF to LSP vector conversion

q_k[i]: the ith coefficient of the kth line spectral pair (LSP) in the cosine domain
k: the two lsf vectors give the LSP vectors q2, q4 at the 2nd and 4th subframes; k = 2*j
f_j[i]: ith coefficient of the jth LSF vector; [0,4000] Hz
f_s: sampling frequency in Hz (8kHz)

Other active modes summary

The process for the other modes is similar to that for the 12.2kbps mode.

indices into code books are parsed from the bit stream
indices give elements of a split matrix quantised (SMQ) residual LSF vector from the relevant code books
prediction from the previous frame is added to obtain the mean-removed LSF vector
the mean is added
the LSF vector is converted to a cosine domain LSP vector

Decoding the SMQ residual LSF vector

The 3 indices are stored with the following numbers of bits:

Mode (kbps)	1st index (bits)	2nd index (bits)	3rd index (bits)
10.2	8	9	9
7.95	9	9	9
7.40	8	9	9
6.70	8	9	9
5.90	8	9	9
5.15	8	8	7
4.75	8	8	7

The four elements of a 'split quantized sub-matrix' are stored at the index position in the appropriate code book are:

1st index in 1st code book: r_1, r_2, r_3
2nd index in 2nd code book: r_4, r_5, r_6
3rd index in 3rd code book: r_7, r_8, r_9, r_10

r_i: residual LSF vector (Hz)
i: the coefficient of vector ( i = 1, ..., 10 )

Mean-removed LSF vector prediction

z_j(n)[i]: the ith coefficient of the mean-removed LSF vector at the jth subframe
r_j(n)[i]: the ith coefficient of the prediction residual vector of frame n at the jth subframe
pred_fac[i]: the ith coefficient of the prediction factor
^r_j(n-1)[i]: the ith coefficient of the the quantified residual vector of the previous frame at the jth subframe

These processes give the LSP vector at the 4th subframe (q4)

LSP vector interpolation (c.f. §5.2.6)

12.2 kbps mode

Other modes

LSP vector to LP filter coefficient conversion (c.f. §5.2.4)

 for i=1..5
   f1[i]  = 2*f1[i-2] - 2*q[2i-1]*f1[i-1]
   for j=i-1..1
     f1[j] +=   f1[j-2] - 2*q[2i-1]*f1[j-1]
   end
 end

f1[-1] = 0; f1[0] = 0;

Same for f2[i] with q[2i] instead of q[2i-1]

 for i=1..5
   f'1[i] = f1[i] + f1[i-1]
   f'2[i] = f2[i] - f2[i-1]
 end

a_i: the LP filter coefficients

Decoding of the pitch (adaptive codebook) vector

indices parsed from bitstream
indices give integer and fractional parts of the pitch lag
pitch vector v(n) is found by interpolating the past excitation u(n) at the pitch lag using an FIR filter. (c.f. §5.6)

Decode pitch lag

Note: division in this section is integer division!

12.2kbps mode - 1/6 resolution pitch lag

First and third subframes

In the first and third subframes, a fractional pitch lag is used with resolutions:

1/6 in the range [17 3/6, 94 3/6]
1 in the range [95, 143]

...encoded using 9 bits.

For [17 3/6, 94 3/6] the pitch index is encoded as:

 pitch_index = (pitch_lag_int - 17)*6 + pitch_lag_frac - 3;

pitch_lag_int: integer part of the pitch lag in the range [17, 94]
pitch_lag_frac: fractional part of the pitch lag in 1/6 units in the range [-2, 3]

so...

 if(pitch_index < (94 4/6 - 17 3/6)*6)
   // fractional part is encoded in range [17 3/6, 94 3/6]
   pitch_lag_int = (pitch_index + 5)/6 + 17;
   pitch_lag_frac = pitch_index - pitch_lag_int*6 + (17 3/6)*6;

And for [95, 143] the pitch index is encoded as:

 pitch_index = (pitch_lag_int - 95) + (94 4/6 - 17 3/6)*6;

pitch_lag_int: integer pitch lag in the range [95, 143]

so...

 else
   // only integer part encoded in range [95, 143], no fractional part
   pitch_lag_int  = pitch_index - (94 4/6 - 17 3/6)*6 + 95;
   pitch_lag_frac = 0;

Second and fourth subframes

In the second and fourth subframes, a pitch lag resolution of 1/6 is always used in the range [T1 - 5 3/6, T1 + 4 3/6], where T1 is nearest integer to the fractional pitch lag of the previous (1st or 3rd) subframe. The search range is bounded by [18, 143]. In this case the pitch delay is encoded using 6 bits and is therefore in the range [0,63].

So the search range for the pitch lag is:

 search_range_min = max(pitch_lag_int_prev - 5, 18);
 search_range_max = search_range_min + 9;
 if(search_range_max > 143) {
   search_range_max = 143;
   search_range_min = search_range_max - 9;
 }

pitch_lag_int_prev: the integer part of the pitch lag from the previous sub frame

The pitch index is encoded as:

 pitch_index = (pitch_lag_int - (search_range_min - 1))*6 + pitch_lag_frac - 3;

pitch_lag_int: the integer part of the pitch lag in the range [search_range_min - 1, search_range_max]
pitch_lag_frac: the fractional part of the pitch lag in the range [-2, 3]

The formula for the pitch_index has been chosen to map pitch_lag_int [search_range_min - 1, search_range_max] and pitch_lag_frac [-2, 3] to [0,60]. (pitch_index = [0, 10]*6 + [-2, 3] - 3 = [0, 6, ..., 60] + [-5, 0] = [0,60])

So the pitch lag is calculated through:

 // integer part of pitch lag = position in range [search_range_min - 1, search_range_max] + lower bound of range
 pitch_lag_int  = (pitch_index + 5)/6 + search_range_min - 1;
 // fractional part of pitch lag = pitch index - (integer part without offset)*6 - 1 3/6 offset to bring the values to the correct range
 pitch_lag_frac = pitch_index - ((pitch_index + 5)/6)*6 - 9;

Note that when using integers and integer division to conduct (pitch_index + 5)/6 the result is similar to taking the ceiling of pitch_index/6.0.

Others modes - 1/3 resolution pitch lag

First and third subframes

In the first and third subframes, a fractional pitch lag is used with resolutions:

1/3 in the range [19 1/3, 84 2/3]
1 in the range [85, 143]

...encoded using 8 bits.

For [19 1/3, 84 2/3] the pitch lag is encoded as:

 pitch_index = pitch_lag_int*3 + pitch_lag_frac - (19 1/3)*3;

pitch_lag_int: integer part of the pitch lag in the range [19, 84]
pitch_lag_frac: fractional part of the pitch lag in 1/3 units in the range [0, 2]

so...

 if(pitch_index < (85 - 19 1/3)*3)
   // fractional part is encoded in range [19 1/3, 84 2/3]
   pitch_lag_int = (pitch_index + 2)/3 + 19;
   pitch_lag_frac = pitch_index - pitch_lag_int*3 + (19 1/3)*3;

And for [85, 143] the pitch index is encoded as:

 pitch_index = pitch_lag_int - 85 + (85 - 19 1/3)*3;

pitch_lag_int: integer pitch lag in the range [85, 143]

so...

 else
   // only integer part encoded in range [85, 143], no fractional part
   pitch_lag_int  = pitch_index - (85 - 19 1/3)*3 + 85;
   pitch_lag_frac = 0;

Second and fourth subframes

In the second and fourth subframes, the pitch lag resolution varies depending on the mode as follows:

7.95 kbps mode
- resolution of 1/3 is always used in the range [T1 - 10 2/3, T1 + 9 2/3]
- encoded using 6 bits => pitch_index is in the range [0, 63]
10.2 and 7.40 kbps modes
- resolution of 1/3 is always used in the range [T1 - 5 2/3, T1 + 4 2/3]
- encoded using 5 bits => pitch_index is in the range [0, 31]
6.70, 5.90, 5.15 and 4.75 kbps modes
- resolution of 1 is used in the range [T1 - 5, T1 + 4]
- resolution of 1/3 is always used in the range [T1 - 1 2/3, T1 + 2/3]
- encoded using 4 bits => pitch_index is in the range [0, 15]

Where T1 is nearest integer to the fractional pitch lag of the previous (1st or 3rd) subframe. The search range is bounded by [20, 143].

So the search range for the pitch lag is:

 lower_bound = 5;
 range = 9;
 if(mode == 7.95) {
   lower_bound = 10;
   range = 19;
 }
 search_range_min = max(pitch_lag_int_prev - lower_bound, 20);
 search_range_max = search_range_min + range;
 if(search_range_max > 143) {
   search_range_max = 143;
   search_range_min = search_range_max - range;
 }

pitch_lag_int_prev: the integer part of the pitch lag from the previous sub frame

For modes 7.40, 7.95 and 10.2 the pitch index is encoded as:

 pitch_index = (pitch_lag_int - search_range_min)*3 + pitch_lag_frac + 2;

pitch_lag_int: the integer part of the pitch lag in the range [search_range_min, search_range_max]
pitch_lag_frac: the fractional part of the pitch lag in the range [-1, 1]

So the pitch lag is calculated through:

 // integer part of pitch lag = position of pitch lag in range [search_range_min, search_range_max] + lower bound of the range
 pitch_lag_int = (pitch_index + 2)/3 - 1 + search_range_min;
 // fractional part of pitch lag = pitch index - (integer part without offset)*3 - 2/3 to bring the values to the correct range
 pitch_lag_frac = pitch_index - ((pitch_index + 2)/3 - 1)*3 - 2;

For modes 4.75, 5.15, 5.90 and 6.70:

 t1_temp = max( min(pitch_lag_int_prev, search_range_min + 5), search_range_max - 4 );

t1_temp: predicted pitch lag from the previous frame adjusted to fit into the 0 position of the search range

The pitch index is encoded as:

 // if pitch lag is below T1 - 1 2/3
 if( pitch_lag_int*3 + pitch_lag_frac <= (t1_temp - 2)*3 ) {
   // encode with resolution 1
   index = (pitch_lag_int - t1_temp) + 5;
 // else if pitch lag is below T1 + 1
 }else if( pitch_lag_int*3 + pitch_lag_frac < (t1_temp + 1)*3 ) {
   // encode with resolution 1/3
   index = ( pitch_lag_int*3 + pitch_lag_frac - (t1_temp - 2)*3 ) + 3;
 // else pitch lag is above T1 + 2/3
 }else {
   // encode with resolution 1
   index = (pitch_lag_int - t1_temp) + 11;
 }

pitch_lag_int: the integer part of the pitch lag in the range [search_range_min, search_range_max]
pitch_lag_frac: the fractional part of the pitch lag in the range [-1, 1]

The possible pitch indices and values are:

0	1	2	3	4	5	6	7	8	9	10	11	12	13	14	15
-5	-4	-3	-2	-1 2/3	-1 1/3	-1	-2/3	-1/3	0	1/3	2/3	1	2	3	4

So the pitch lag is calculated through:

 if(pitch_index < 4) {
   // integer part of pitch lag = pitch lag position in range [t1_temp - 5, t1_temp - 2] + lower bound of range
   pitch_lag_int = pitch_index + (t1_temp - 5);
   // this range is coded with resolution 1 so no fractional part
   pitch_lag_frac = 0;
 }else if(pitch_index < 12) {
   pitch_lag_int = (pitch_index - 2)/3 + (t1_temp - 2);
   pitch_lag_frac = (pitch_index - 4) - ((pitch_index - 2)/3)*3 - 11;
 }else {
   // integer part of pitch lag = pitch lag position in range [t1_temp + 1, t1_temp + 4] + lower bound of range
   pitch_lag_int = pitch_index - 12 + t1_temp + 1;
   // this range is coded with resolution 1 so no fractional part
   pitch_lag_frac = 0;
 }

Calculate pitch vector

k: integer pitch lag
n: sample position in the vectors 0, ..., 39
t: 0, ..., 5 corresponding to fractions 0, 1/6, 2/6, 3/6, -2/6, -1/6 respectively

This equation can be used for both 1/3 and 1/6 resolution simply by multiplying t by 2 in the 1/3 case.

(Note: the coefficients b60 are in the reference source in an array called inter6)

Decoding of the fixed (innovative or algebraic) vector

the excitation pulse positions and signs are parsed from the bit stream
the pulse positions and signs are encoded differently depending on the mode
the fixed code book vector, c(n), is then constructed from the pulse positions and signs
if pitch_lag_int is less than the subframe size (40), the pitch sharpening procedure is applied

Decoding the pulse positions

12.2 kbps mode

10 pulse positions each coded using 3 bit Gray codes
signs coded using 1 bit each for 5 pulse pairs

Pulse	Positions
i0,i5	0, 5, 10, 15, 20, 25, 30, 35
i1,i6	1, 6, 11, 16, 21, 26, 31, 36
i2,i7	2, 7, 12, 17, 22, 27, 32, 37
i3,i8	3, 8, 13, 18, 23, 28, 33, 38
i4,i9	4, 9, 14, 19, 24, 29, 34, 39

10.2 kbps mode

8 pulse positions, 4 pairs, coded as 3 values using 10, 10 and 7 bits
signs coded using 1 bit each for 4 pulse pairs

Pulse	Positions
i0,i4	0, 4, 8, 12, 16, 20, 24, 28, 32, 36
i1,i5	1, 5, 9, 13, 17, 21, 25, 29, 33, 37
i2,i6	2, 6, 10, 14, 18, 22, 26, 30, 34, 38
i3,i7	3, 7, 11, 15, 19, 23, 27, 31, 35, 39

7.95 and 7.40 kbps modes

4 pulse positions Gray coded using 3, 3, 3 and 4 bits
signs coded using 1 bit for each pulse

Pulse	Positions
i0	0, 5, 10, 15, 20, 25, 30, 35
i1	1, 6, 11, 16, 21, 26, 31, 36
i2	2, 7, 12, 17, 22, 27, 32, 37
i3	3, 8, 13, 18, 23, 28, 33, 38 4, 9, 14, 19, 24, 29, 34, 39

6.70 kbps mode

3 pulse positions coded using 3, 4 and 4 bits
signs coded using 1 bit for each pulse

Pulse	Positions
i0	0, 5, 10, 15, 20, 25, 30, 35
i1	1, 6, 11, 16, 21, 26, 31, 36 3, 8, 13, 18, 23, 28, 33, 38
i2	2, 7, 12, 17, 22, 27, 32, 37 4, 9, 14, 19, 24, 29, 34, 39

5.90 kbps mode

2 pulse positions coded using 4 and 5 bits
signs coded using 1 bit for each pulse

Pulse	Positions
i0	1, 6, 11, 16, 21, 26, 31, 36 3, 8, 13, 18, 23, 28, 33, 38
i1	0, 5, 10, 15, 20, 25, 30, 35 1, 6, 11, 16, 21, 26, 31, 36 2, 7, 12, 17, 22, 27, 32, 37 4, 9, 14, 19, 24, 29, 34, 39

5.15 and 4.75 kbps modes

2 pulse positions coded using 1 bit for the position subset and 3 bits per pulse
signs coded using 1 bit for each pulse

Subframe	Subset	Pulse	Positions
1	1	i0	0, 5, 10, 15, 20, 25, 30, 35
	1	i1	2, 7, 12, 17, 22, 27, 32, 37
	2	i0	1, 6, 11, 16, 21, 26, 31, 36
	2	i1	3, 8, 13, 18, 23, 28, 33, 38
2	1	i0	0, 5, 10, 15, 20, 25, 30, 35
	1	i1	3, 8, 13, 18, 23, 28, 33, 38
	2	i0	2, 7, 12, 17, 22, 27, 32, 37
	2	i1	4, 9, 14, 19, 24, 29, 34, 39
3	1	i0	0, 5, 10, 15, 20, 25, 30, 35
	1	i1	2, 7, 12, 17, 22, 27, 32, 37
	2	i0	1, 6, 11, 16, 21, 26, 31, 36
	2	i1	4, 9, 14, 19, 24, 29, 34, 39
4	1	i0	0, 5, 10, 15, 20, 25, 30, 35
	1	i1	3, 8, 13, 18, 23, 28, 33, 38
	2	i0	1, 6, 11, 16, 21, 26, 31, 36
	2	i1	4, 9, 14, 19, 24, 29, 34, 39

Fixed codebook vector construction

All c(n) are zero if there is no pulse at position n. If there is a pulse at position n then it has the corresponding sign as parsed above.

Pitch sharpening

β: the decoded pitch gain, ^g_p, bounded by [0.0,1.0] for 12.2. kbps or [0.0,0.8] for other modes

Note: Only sharpen n in [pitch_lag_int-1, 39]. The reference source considers previous fixed vector elements (n<0) to be 0. I think this is also the reason pitch sharpening is not conducted for pitch_lag_int > 40 as all the sharpening contributions would be 0.

Decoding of the pitch and fixed codebook gains

Fixed gain prediction

A moving average prediction of the innovation (fixed) energy is conducted.

g_c': fixed gain prediction
\tilde{E}: predicted energy [dB]
\bar{E}: desired mean innovation (fixed) energy [dB]
E_I: calculated mean innovation (fixed) energy [dB]

b: 4-tap MA prediction coefficients [0.68, 0.58, 0.34, 0.19]
^R(k): quantified prediction errors at subframe k 20*log10(^γ_gc(k))

Desired mean innovation (fixed) energy:

Mode (kbps)	Mean energy (dB)
12.2	36
10.2	33
7.95	36
7.40	30
6.70	28.75
5.90	33
5.15	33
4.75	33

E_I: calculated mean innovation (fixed) energy [dB]
N: subframe size 40
c(n): fixed codebook vector

Dequantisation of the gains

12.2kbps and 7.95kbps - scalar quantised gains

The received indices are used to find the quantified pitch gain, ^g_p, and the quantified fixed gain correction factor, ^γ_gc.

Pitch gain

The parsed gain index is used to obtain the quantified pitch gain, ^g_p, from the corresponding codebook. (qua_gain_pitch in the reference source.)

Fixed gain correction factor

The parsed gain index is used to obtain the quantified fixed gain, ^g_c, from the corresponding codebook. (qua_gain_code in the reference source.) The table stores ^γ_gc, and the quantised energy error in two forms (these are needed for the moving average calculation of the predicted fixed gain):

qua_ener_MR122 = log2(^γ_gc) qua_ener = 20*log10(^γ_gc)

^γ_gc is stored at Q11 (i.e. it's multiplied by 2^11.) qua_ener_MR122 and qua_ener are stored at Q10.

Other modes - vector quantised gains

The received index gives both the quantified adaptive codebook gain, ^g_p, and the quantified algebraic codebook gain correction factor, ^γ_gc.

The tables contains the following data:

^g_p           (Q14),
^γ_gc          (Q12), (^g_c = g_c'*^γ_gc),
qua_ener_MR122 (Q10), (log2(^γ_gc))
qua_ener       (Q10)  (20*log10(^γ_gc))

The log2() and log10() values are calculated on the fixed point value
(g_fac Q12) and not on the original floating point value of g_fac
to make the quantizer/MA predictdor use corresponding values.

The codebook used depends on the mode:

6.70, 7.40, 10.2 kbps modes - table_gain_highrates in the reference source Four consecutive entries give ^g_p, ^γ_gc, qua_ener_MR122 and qua_ener. Apparently the values for qua_ener are the original ones from IS641 to ensure bit-exactness but are not exactly the rounded value of 20log10(^γ_gc).

5.15, 5.90 kbps modes - table_gain_lowrates Similar to table_gain_highrates, four consecutive entries give ^g_p, ^γ_gc, qua_ener_MR122 and qua_ener. There are no special notes for this table.

4.75 kbps mode - table_gain_MR475 Unlike the above mentioned tables, four consecutive values give ^g_p, ^γ_gc for subframes 0,2, and then ^g_p, ^γ_gc for subframes 1,3.

At the very least I think these tables could be redesigned a little.

Calculation of the quantified fixed gain

Smoothing of the fixed codebook gain

10.2, 6.70, 5.90, 5.15, 4.75 kbit/s modes only

Calculate averaged LSP vector

\bar{q}(n): averaged LSP vector at frame n
^q_4(n): quantified LSP vector for the 4th subframe at frame n

Calculate fixed gain smoothing factor

diff_m: difference measure at subframe m
j: loops over LSPs
m: loops over subframes
^q_m: quantified LSP vector at subframe m

Note: I think the sum over the subframes is an error in the specification and they actually mean to use the quantified LSP vector from subframe m for the calculation of diff for subframe m. I think this is what they do in the reference source and the sum over m is an error.

k_m: fixed gain smoothing factor
K_1: 0.4
K_2: 0.25
diff_m: difference measure at subframe m

If diff_m has been greater than 0.65 for 10 subframes, k_m is set to 1.0 (i.e. no smoothing) for 40 subframes.

Calculate mean fixed gain

\bar{g_c}(m): mean fixed gain at subframe m
^g_c(k): quantified fixed gain at subframe k

Calculate smoothed fixed gain

^g_c: quantified fixed gain
\bar{g_c}: averaged fixed gain

Anti-sparseness processing

7.95, 6.70, 5.90, 5.15, 4.75 kbit/s modes only

Evaluate impulse response filter strength

The fixed vector, c(n), has only a few pulses per subframe. In certain conditions, to reduce perceptual artifacts arising from this, the vector is circularly convolved with a predefined impulse response. The selection of the strength of filter is made based on the decoded gains.

 if ^g_p < 0.6
   impNr = 0
 else if ^g_p < 0.9
   impNr = 1
 else
   impNr = 2

impNr = 0: strong impulse response filter
impNr = 1: medium impulse response filter
impNr = 2: no filtering

 if ^g_c(k) > 2 * ^g_c(k-1)
   impNr = min( impNr + 1, 2 )
 else if impNr = 0 AND median of last five ^g_p >= 0.6
   impNr = min( impNr(k), min(impNr(k-1) + 1, 2) )

Circular convolution of fixed vector and impulse response filter

(c * h): convolution of vectors c and h, in this case circular convolution
h[n]: nth coefficient of the impulse response used for filtering
c[n]: nth coefficient of the fixed vector

n = 0, ..., 39

To make the convolution circular, make the impulse response circular by taking h[-m] = h[39-m]

Computing the reconstructed speech

Construct excitation

u(n): excitation vector
^g_p: quantified pitch gain
v(n): pitch vector
^g_c: quantified fixed gain
c(n): fixed vector

Emphasise pitch vector contribution

This is apparently a post-processing technique.

^u(n): excitation vector with emphasised pitch vector contribution
β: ^g_p bounded by [0.0, 0.8] or [0.0, 1.0] depending on mode

Apply adaptive gain control (AGC) through gain scaling

η: gain scaling factor for emphasised excitation

^u'(n): gain-scaled emphasised excitation

Calculate reconstructed speech samples

^s(n): reconstructed speech samples
^a_i: LP filter coefficients

Note: for n-i < 0, ^s(n-i) should be take from previous speech samples if existing, else we will consider them 0 as this behaviour is undefined the specification.

Additional instability protection

If an overflow occurs during synthesis, the pitch vector, v(n), is scaled down by a factor of 4 and synthesis is conducted again bypassing emphasising the pitch vector contribution and adaptive gain control.

Q: What classifies an overflow? A: s(n) < -32768 or s(n) > 32767 (i.e. 16-bit signed int)

Post-processing

Adaptive post-filtering

(c.f. §6.2.1)

IIR filtering

The speech samples, ^s(n), are filtered through a formant filter and a tilt compensation filter.

γ_n, γ_d: control the amount of formant post-filtering

The speech samples, ^s(n), are filtered through ^A(z/γ_n) to produce the residual signal ^r(n).

^r(n) is filtered through 1/^A(z/γ_d).

The output is filtered through H_t(z) (the tilt compensation filter) resulting in the post-filtered speech signal, ^s_f(n).

L_h: 22 - the truncation of the impulse response
h_f: impulse response of the formant filter H_f

So, in summation notation:

12.2 and 10.2 kbps modes

γ_n = 0.7

γ_d = 0.75

γ_t = 0.8 if k_1' > 0; 0 otherwise

Other modes

γ_n = 0.55

γ_d = 0.7

γ_t = 0.8

Adaptive gain control

Adaptive gain control is used to compensate for the gain difference between the filtered and synthesised speech signals.

\alpha: adaptive gain control factor equal to 0.9

High-pass filtering and upscaling

(c.f. §6.2.2)

After completing all filtering, the samples are scaled up by a factor of 2.

AMR-NB

Nomenclature weirdness

Summary

Bit stream frame format

IF1 format

IF2 format

GSM OTA format

Field meaning

Classes

Decoding of LP filter parameters

12.2kbps mode summary

Decoding SMQ residual LSF vectors

Mean-removed LSF vector prediction

The mean is added

LSF to LSP vector conversion

Other active modes summary

Decoding the SMQ residual LSF vector

Mean-removed LSF vector prediction

LSP vector interpolation (c.f. §5.2.6)

12.2 kbps mode

Other modes

LSP vector to LP filter coefficient conversion (c.f. §5.2.4)

Decoding of the pitch (adaptive codebook) vector

Decode pitch lag

12.2kbps mode - 1/6 resolution pitch lag

First and third subframes

Second and fourth subframes

Others modes - 1/3 resolution pitch lag

First and third subframes

Second and fourth subframes

Calculate pitch vector

Decoding of the fixed (innovative or algebraic) vector

Decoding the pulse positions

12.2 kbps mode

10.2 kbps mode

7.95 and 7.40 kbps modes

6.70 kbps mode

5.90 kbps mode

5.15 and 4.75 kbps modes

Fixed codebook vector construction

Pitch sharpening

Decoding of the pitch and fixed codebook gains

Fixed gain prediction

Dequantisation of the gains

12.2kbps and 7.95kbps - scalar quantised gains

Pitch gain

Fixed gain correction factor

Other modes - vector quantised gains

Calculation of the quantified fixed gain

Smoothing of the fixed codebook gain

Calculate averaged LSP vector

Calculate fixed gain smoothing factor

Calculate mean fixed gain

Calculate smoothed fixed gain

Anti-sparseness processing

Evaluate impulse response filter strength

Circular convolution of fixed vector and impulse response filter

Computing the reconstructed speech

Construct excitation

Emphasise pitch vector contribution

Apply adaptive gain control (AGC) through gain scaling

Calculate reconstructed speech samples

Additional instability protection

Post-processing

Adaptive post-filtering

IIR filtering

12.2 and 10.2 kbps modes

Other modes

Adaptive gain control

High-pass filtering and upscaling

Navigation menu

Search